Understanding Matrices And Matrix Notation
Understanding Matrices And Matrix Notation Youtube In order to do linear algebra, we will have to know how to use matrices. so what's a matrix? it's just an array of numbers listed in a grid of particular dim. To add two matrices: add the numbers in the matching positions: these are the calculations: 3 4=7. 8 0=8. 4 1=5. 6−9=−3. the two matrices must be the same size, i.e. the rows must match in size, and the columns must match in size. example: a matrix with 3 rows and 5 columns can be added to another matrix of 3 rows and 5 columns.

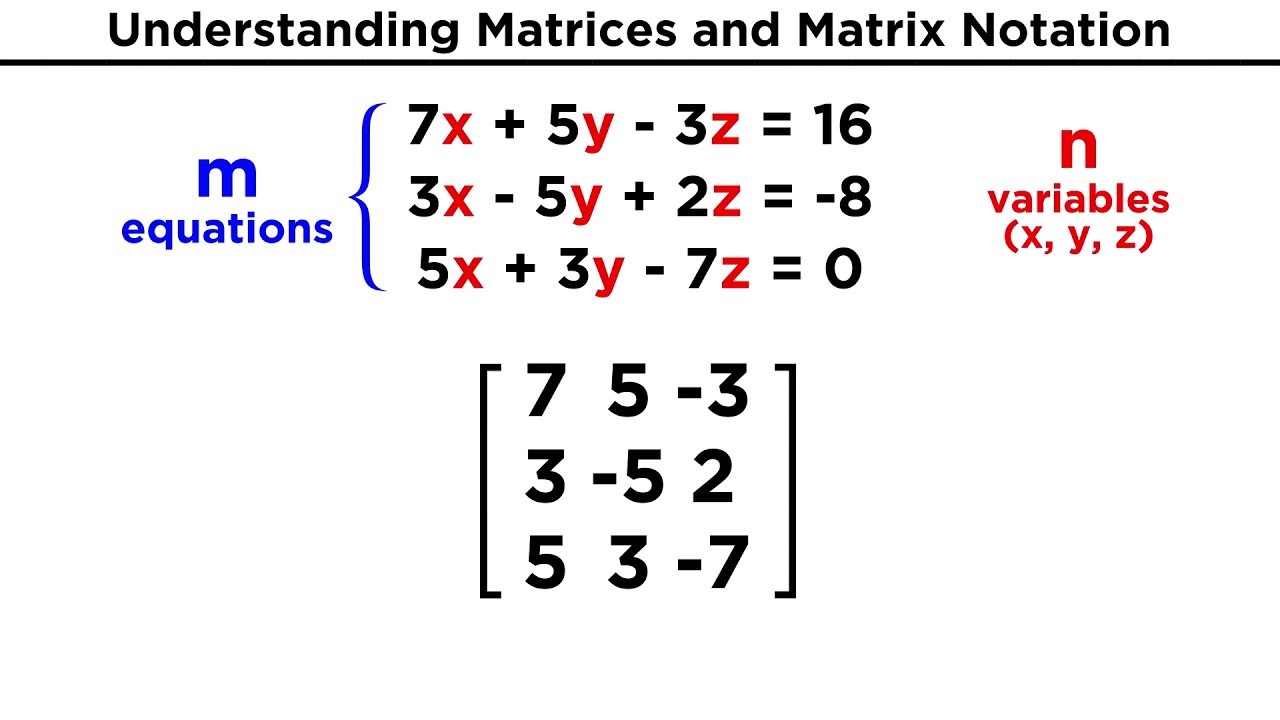
Matrices Basics Definition Notation Order Youtube This page titled 5.4: matrix notation is shared under a cc by nc 4.0 license and was authored, remixed, and or curated by dirk colbry via source content that was edited to the style and standards of the libretexts platform. A row in a matrix is a set of numbers that are aligned horizontally. a column in a matrix is a set of numbers that are aligned vertically. each number is an entry, sometimes called an element, of the matrix. matrices (plural) are enclosed in [ ] or ( ), and are usually named with capital letters. for example, three matrices named a, b, and c. Note that in aij, we write the row number i. before the column number j. an m. 1 matrix is a column vector with m rows and 1 column. 1. matrix is a row vector with 1 row and n columns. the m n matrix a consists of: n columns in the form of m vectors aj = (aij)m 2 m i=1 r for j = 1; 2; : : : ; n;. What is a matrix. in mathematics, a matrix (plural matrices) is a rectangular array of numbers, symbols, or expressions, arranged in rows and columns. matrices are commonly written in box brackets. the horizontal and vertical lines of entries in a matrix are called rows and columns, respectively. the size of a matrix is defined by the number of.

What Is Matrix Notation In Math Studypug Note that in aij, we write the row number i. before the column number j. an m. 1 matrix is a column vector with m rows and 1 column. 1. matrix is a row vector with 1 row and n columns. the m n matrix a consists of: n columns in the form of m vectors aj = (aij)m 2 m i=1 r for j = 1; 2; : : : ; n;. What is a matrix. in mathematics, a matrix (plural matrices) is a rectangular array of numbers, symbols, or expressions, arranged in rows and columns. matrices are commonly written in box brackets. the horizontal and vertical lines of entries in a matrix are called rows and columns, respectively. the size of a matrix is defined by the number of. Matrix notation. this page summarizes the notation commonly used when working with matrices. matrix dimensions. whenever we say "a is an m by n matrix," or simply "a is m x n," for some positive integers m and n, this means that a has m rows and n columns. matrix a below is an example of a 3 x 5 (three by five) matrix: vectors. Learn about matrix notation, dimension, addition, and scalar multiplication. optionally, learn the formula for matrix multiplication.

Comments are closed.