Second Order Differential Equations Teaching Resources

2nd Order Differential Equations Teaching Resources File previews. pptx, 1.07 mb. this resource is designed to deliver 2nd order differential equations as part of the core mathematics 2 section of the further mathematics a level curriculum. it is a powerpoint which covers homogeneous and non homogeneous 2nd order equations with and without boundary conditions. creative commons "sharealike". A 'teach further maths' resource. 78 slides to understand what is meant by a ‘second order differential equation’. to be able to solve some second order differential equations using the auxiliary equation. to be able to solve some second order differential equations by finding a complementary function and a particular integral.
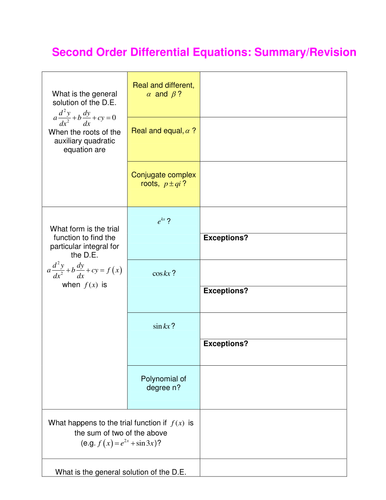
Second Order Differential Equations Teaching Resources 5 questions on second order differential equations. first two and last, linear with constant coefficients; first two homogeneous, complex and repeated roots. last, non homogeneous. third, motion under gravity. fourth, linear with a given particular solution (variation of parameters). numbas resources have been made available under a creative. The motion of a spring. in additional topics: applications of second order differential equations we will further pursue this application as well as the application to electric circuits. in this section we study the case where , for all , in equation 1. such equa tions are called homogeneous linear equations. thus, the form of a second order. Ordinary differential equations lecture notes, lecture slides, exercises, maple worksheets, etc. introduction: classification of differential equations (orders, single eqs vs systems, linear vs nonlinear): slides. direction fields: maple. first order scalar differential equations: separable equations: dy dt=f (t)g (y). 5 questions on second order differential equations. first two and last, linear with constant coefficients; first two homogeneous, complex and repeated roots. last, non homogeneous. third, motion under gravity. fourth, linear with a given particular solution (variation of parameters). numbas resources have been made available under a creative.

Second Order Differential Equations Teaching Resources Ordinary differential equations lecture notes, lecture slides, exercises, maple worksheets, etc. introduction: classification of differential equations (orders, single eqs vs systems, linear vs nonlinear): slides. direction fields: maple. first order scalar differential equations: separable equations: dy dt=f (t)g (y). 5 questions on second order differential equations. first two and last, linear with constant coefficients; first two homogeneous, complex and repeated roots. last, non homogeneous. third, motion under gravity. fourth, linear with a given particular solution (variation of parameters). numbas resources have been made available under a creative. Teach yourself (1) second order differential equations: a summary of how to solve second order odes with constant coefficients: how to solve homogeneous equations (with no right hand side) and how to solve equations with a right hand side (method of undetermined coefficients and methods of variation of parameters). To solve a linear second order differential equation of the form. d 2 ydx 2 p dydx qy = 0. where p and q are constants, we must find the roots of the characteristic equation. r 2 pr q = 0. there are three cases, depending on the discriminant p 2 4q. when it is. positive we get two real roots, and the solution is. y = ae r 1 x be r 2 x.
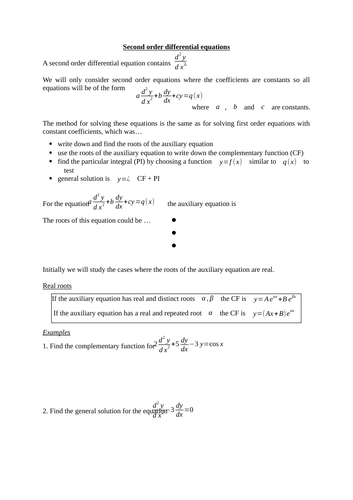
Second Order Differential Equations Worksheet Teaching Resources Teach yourself (1) second order differential equations: a summary of how to solve second order odes with constant coefficients: how to solve homogeneous equations (with no right hand side) and how to solve equations with a right hand side (method of undetermined coefficients and methods of variation of parameters). To solve a linear second order differential equation of the form. d 2 ydx 2 p dydx qy = 0. where p and q are constants, we must find the roots of the characteristic equation. r 2 pr q = 0. there are three cases, depending on the discriminant p 2 4q. when it is. positive we get two real roots, and the solution is. y = ae r 1 x be r 2 x.

Comments are closed.