Second Order Differential Equations 2

2nd Order Differential Equations Teaching Resources Definition: characteristic equation. the characteristic equation of the second order differential equation ay'' by' cy=0 is. a\lambda^2 b\lambda c=0. \nonumber. the characteristic equation is very important in finding solutions to differential equations of this form. To solve a linear second order differential equation of the form. d 2 ydx 2 p dydx qy = 0. where p and q are constants, we must find the roots of the characteristic equation. r 2 pr q = 0. there are three cases, depending on the discriminant p 2 4q. when it is. positive we get two real roots, and the solution is. y = ae r 1 x be r 2 x.

Second Order Linear Differential Equations Youtube Let us consider a few examples of each type to understand how to determine the solution of the homogeneous second order differential equation. example 1: solve the 2nd order differential equation y'' 6y' 5y = 0. solution: assume y = e rx and find its first and second derivative: y' = re rx, y'' = r 2 e rx. The general solution of a homogeneous linear second order equation. if y1 and y2 are defined on an interval (a, b) and c1 and c2 are constants, then. y = c1y1 c2y2. is a linear combination of y1 and y2. for example, y = 2cosx 7sinx is a linear combination of y1 = cosx and y2 = sinx, with c1 = 2 and c2 = 7. Using the characteristic equation to solve second order differential equations with constant coefficients. write the differential equation in the form a y ″ b y ′ c y = 0. a y ″ b y ′ c y = 0. find the corresponding characteristic equation a λ 2 b λ c = 0. a λ 2 b λ c = 0. either factor the characteristic equation or. Repeated roots – in this section we discuss the solution to homogeneous, linear, second order differential equations, ay′′ by′ cy = 0 a y ″ b y ′ c y = 0, in which the roots of the characteristic polynomial, ar2 br c = 0 a r 2 b r c = 0, are repeated, i.e. double, roots. we will use reduction of order to derive the second.

Solving Second Order Differential Equations Youtube Using the characteristic equation to solve second order differential equations with constant coefficients. write the differential equation in the form a y ″ b y ′ c y = 0. a y ″ b y ′ c y = 0. find the corresponding characteristic equation a λ 2 b λ c = 0. a λ 2 b λ c = 0. either factor the characteristic equation or. Repeated roots – in this section we discuss the solution to homogeneous, linear, second order differential equations, ay′′ by′ cy = 0 a y ″ b y ′ c y = 0, in which the roots of the characteristic polynomial, ar2 br c = 0 a r 2 b r c = 0, are repeated, i.e. double, roots. we will use reduction of order to derive the second. Differential equations 3 units · 8 skills. unit 1 first order differential equations. unit 2 second order linear equations. unit 3 laplace transform. Second order constant coefficient differential equations can be used to model spring mass systems. an examination of the forces on a spring mass system results in a differential equation of the form \[mx″ bx′ kx=f(t), \nonumber \] where mm represents the mass, bb is the coefficient of the damping force, \(k\) is the spring constant, and \(f.
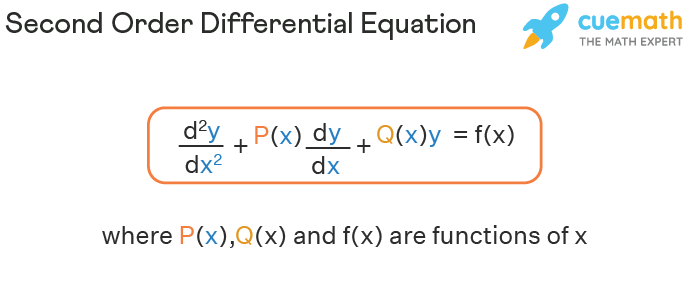
Second Order Differential Equation Solver Types Examples Methods Differential equations 3 units · 8 skills. unit 1 first order differential equations. unit 2 second order linear equations. unit 3 laplace transform. Second order constant coefficient differential equations can be used to model spring mass systems. an examination of the forces on a spring mass system results in a differential equation of the form \[mx″ bx′ kx=f(t), \nonumber \] where mm represents the mass, bb is the coefficient of the damping force, \(k\) is the spring constant, and \(f.

Comments are closed.