Kirchhoff S Law Statement Equations And Diagrams

Kirchhoff S Law Statement Equations And Diagrams Kirchhoff’s current law states that the “total current entering a junction or node is exactly equal to the current leaving the node.”. in other words, the algebraic sum of all the currents entering the node must be equal to the algebraic sum of all the currents leaving a node. this law is commonly known as the conservation of charge. Kirchhoff’s second law or kirchhoff’s voltage law. according to kirchhoff’s voltage law, the voltage around a loop equals the sum of every voltage drop in the same loop for any closed network and equals zero. put differently, the algebraic sum of every voltage in the loop has to be equal to zero and this property of kirchhoff’s law is.

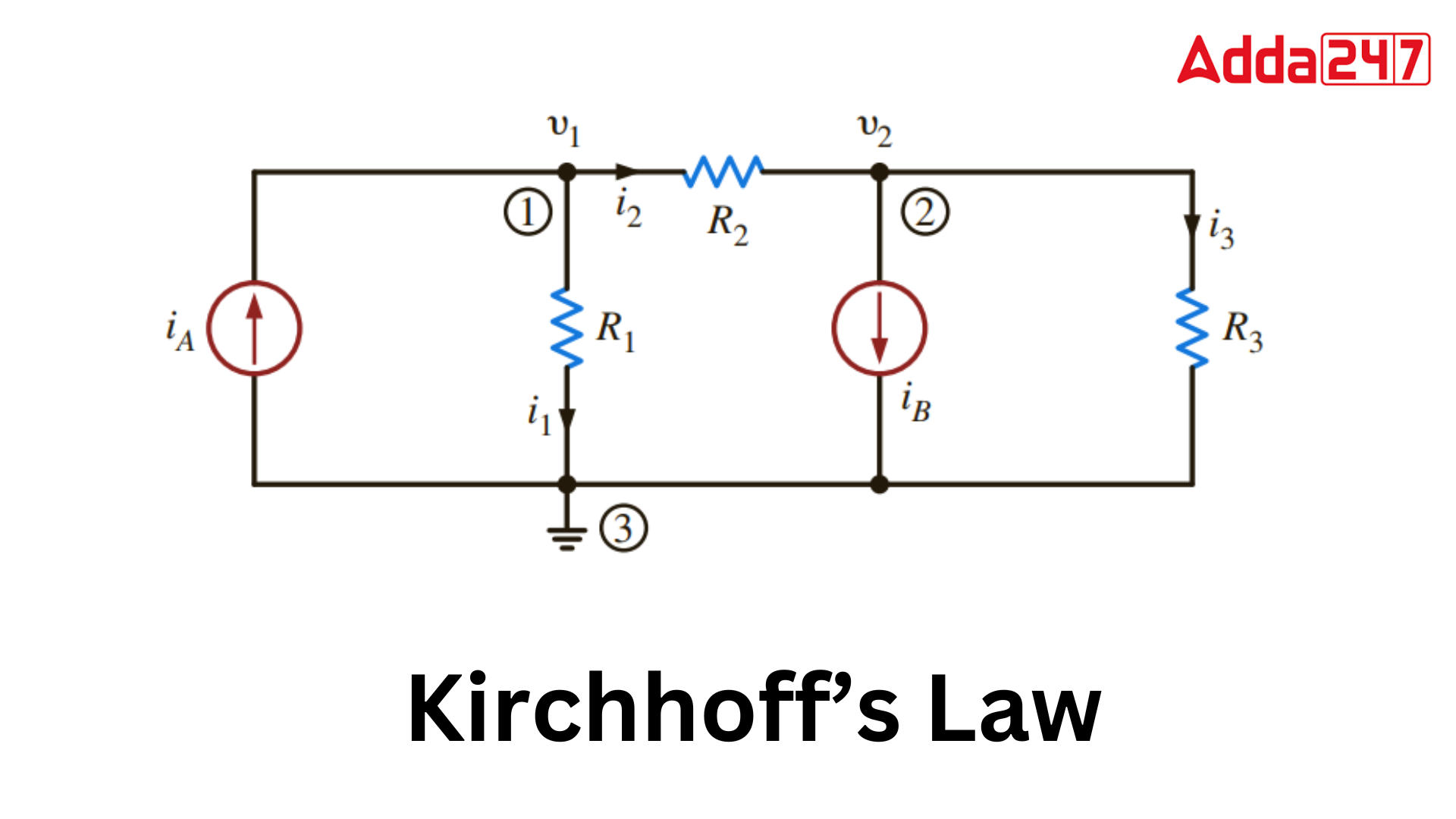
Kirchhoff S Law Statement Equations And Diagrams The sum of all currents entering a junction must equal the sum of all currents leaving the junction: ∑iin = ∑iout. ∑ i i n = ∑ i o u t. (10.4.1) kirchhoff’s second rule—the loop rule. the algebraic sum of changes in potential around any closed circuit path (loop) must be zero: ∑v = 0. ∑ v = 0. (10.4.2). The circuit has 3 branches, 2 nodes (a and b) and 2 independent loops. using kirchhoffs current law, kcl the equations are given as: at node a : i1 i2 = i3. at node b : i3 = i1 i2. using kirchhoffs voltage law, kvl the equations are given as: loop 1 is given as : 10 = r1 i1 r3 i3 = 10i1 40i3. Thus substitution of i3 in terms of i2 gives us the value of i3 as 0.5 amps. as kirchhoff’s junction rule states that : i1 = i2 i3. the supply current flowing through resistor r1 is given as : 1.0 0.5 = 1.5 amps. thus i1 = it = 1.5 amps, i2 = 1.0 amps and i3 = 0.5 amps and from that information we could calculate the i*r voltage drops. Kirchhoff’s current law, often shortened to kcl, states that “the algebraic sum of all currents entering and exiting a node must equal zero.”. this law is used to describe how a charge enters and leaves a wire junction point or node on a wire. armed with this information, let’s now take a look at an example of the law in practice, why.

Kirchhoff S Laws Formula Equation Examples Thus substitution of i3 in terms of i2 gives us the value of i3 as 0.5 amps. as kirchhoff’s junction rule states that : i1 = i2 i3. the supply current flowing through resistor r1 is given as : 1.0 0.5 = 1.5 amps. thus i1 = it = 1.5 amps, i2 = 1.0 amps and i3 = 0.5 amps and from that information we could calculate the i*r voltage drops. Kirchhoff’s current law, often shortened to kcl, states that “the algebraic sum of all currents entering and exiting a node must equal zero.”. this law is used to describe how a charge enters and leaves a wire junction point or node on a wire. armed with this information, let’s now take a look at an example of the law in practice, why. Kirchhoff’s law of current is crucial in both the design and analysis of electrical circuits. engineers and technicians use this law to make sure circuits in our gadgets and appliances function properly and safely. it’s essential in designing circuit boards, helping to manage how current splits and combines in various parts of the circuit. The current entering any junction is equal to the current leaving that junction. i 2 i 3 = i 1 i 4. this law, also called kirchhoff's first law, or kirchhoff's junction rule, states that, for any node (junction) in an electrical circuit, the sum of currents flowing into that node is equal to the sum of currents flowing out of that node; or equivalently:.