Geometry Points Use Soh Cah Toa To Write Sin Cos And Tan As A

Geometry Points Use Soh Cah Toa To Write Sin Cos And Tan As A Decimal Youtube It’s a mnemonic device to help you remember the three basic trig ratios used to solve for missing sides and angles in a right triangle. it’s defined as: soh: sin (θ) = opposite hypotenuse. cah: cos (θ) = adjacent hypotenuse. toa: tan (θ) = opposite adjacent. Sine, cosine and tangent. and sine, cosine and tangent are the three main functions in trigonometry. they are often shortened to sin, cos and tan. the calculation is simply one side of a right angled triangle divided by another side we just have to know which sides, and that is where "sohcahtoa" helps.

Geometry Points Use Soh Cah Toa To Find Tan Youtube Please subscribe: channel uchkkyp6ezvqq5kunzva mlg?sub confirmation=1to use soh cah toa to write the ratios for sine, cosine, and tan. We can use trigonometry to work out the unknown sides of a right angled triangle by using sohcahtoa. label the sides of the right angled triangle that we have information about. choose the trig ratio we need. substitute the values from the triangle into the function. calculate the unknown side, rearranging if necessary. A basic introduction to defining the three major trigonometric functions (sine, cosine, tangent) are discussed using the mnemonic soh cah toa. a review of basic trigonometry: sine, cosine & tangent including how to use the ratios to find missing sides and missing angles on right triangles. students learn that the sine of an angle of a right. We can use sohcahtoa if we know one side and one angle other than the right angle. given the first case where we know two sides, it is also possible to find the third side of the triangle using the pythagorean theorem, a 2 b 2 = c 2. in the case of a right triangle we can replace the variables a, b, and c with o, a, and h (o and a can be.

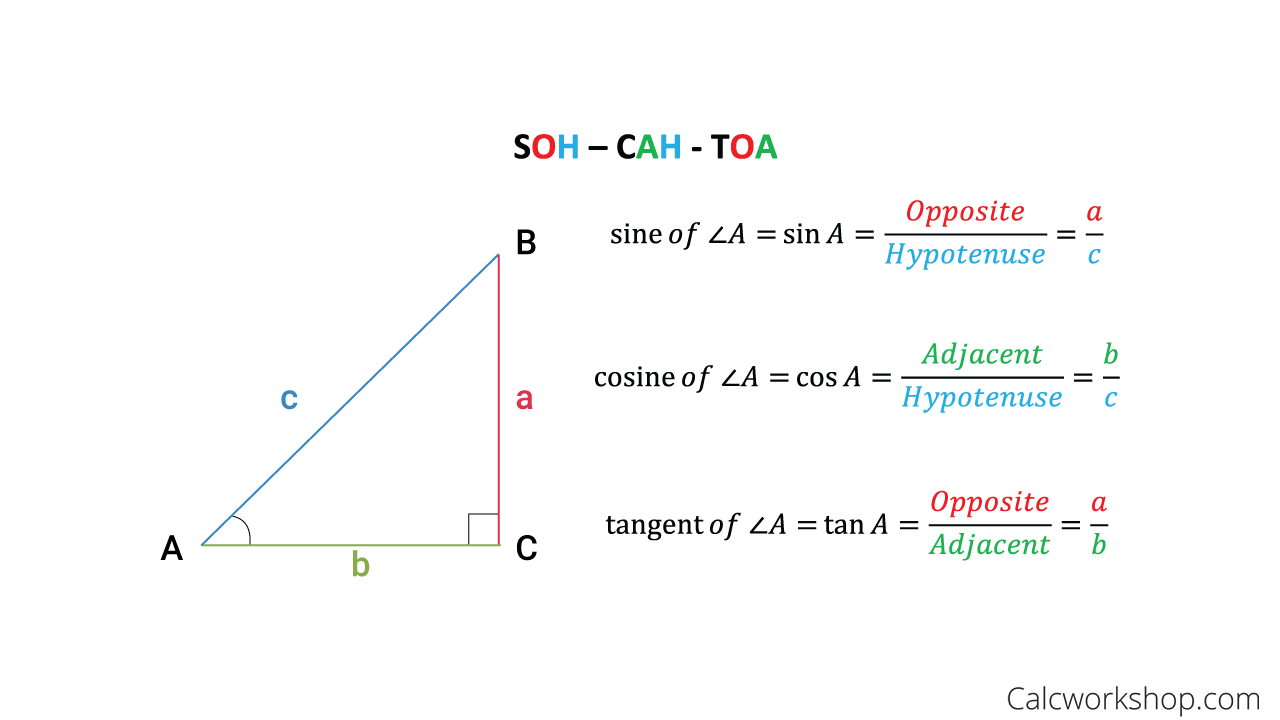
Sohcahtoa Triangle Formula A basic introduction to defining the three major trigonometric functions (sine, cosine, tangent) are discussed using the mnemonic soh cah toa. a review of basic trigonometry: sine, cosine & tangent including how to use the ratios to find missing sides and missing angles on right triangles. students learn that the sine of an angle of a right. We can use sohcahtoa if we know one side and one angle other than the right angle. given the first case where we know two sides, it is also possible to find the third side of the triangle using the pythagorean theorem, a 2 b 2 = c 2. in the case of a right triangle we can replace the variables a, b, and c with o, a, and h (o and a can be. Cah — cosine of angle is adjacent over hypotenuse. toa — tangent of angle is opposite over adjacent. we can use sohcahtoa to create three different equations for finding the value of angle θ in the right triangle like shown below. they are given as: sin (θ) = opposite hypotenuse. cos (θ) = adjacent hypotenuse. tan (θ) = opposite adjacent. "sohcahtoa" is a helpful mnemonic for remembering the definitions of the trigonometric functions sine, cosine, and tangent i.e., sine equals opposite over hypotenuse, cosine equals adjacent over hypotenuse, and tangent equals opposite over adjacent, sintheta = (opposite) (hypotenuse) (1) costheta = (adjacent) (hypotenuse) (2) tantheta = (opposite) (adjacent). (3) other mnemonics include 1.

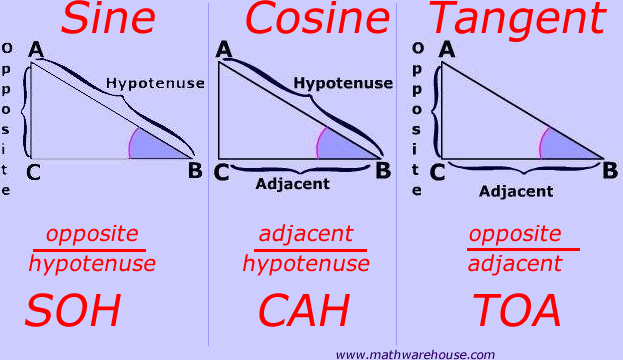
Sine Cosine Tangent Explained And With Examples And Practice Identifying Opposite Adjacent Cah — cosine of angle is adjacent over hypotenuse. toa — tangent of angle is opposite over adjacent. we can use sohcahtoa to create three different equations for finding the value of angle θ in the right triangle like shown below. they are given as: sin (θ) = opposite hypotenuse. cos (θ) = adjacent hypotenuse. tan (θ) = opposite adjacent. "sohcahtoa" is a helpful mnemonic for remembering the definitions of the trigonometric functions sine, cosine, and tangent i.e., sine equals opposite over hypotenuse, cosine equals adjacent over hypotenuse, and tangent equals opposite over adjacent, sintheta = (opposite) (hypotenuse) (1) costheta = (adjacent) (hypotenuse) (2) tantheta = (opposite) (adjacent). (3) other mnemonics include 1.